3rd example

project <Β_422-3>

The structure of this example derives from the 1st example by removing beams b1, b2 and appending a cantilever at the side of beams b5 and b6.

The thickness of all slabs is 190 mm satisfying the slenderness. S1, S2 are three-edge-supported slabs. Slab s5 is cantilever, carrying additional concentrated linear dead load G=1.0 kN/m on its free edges. The cantilever is fixed along the small side of the two-way slabs s3 and s4. To per-form analysis set these parameters: Tab “Meshing”: “Overall size” = 0.10 m, “Perimeter size” = 0.05 m, Tab “Modules”: “SLABS” = ON, Tab “Loads”: “Adverse Slabs” = ΟΝ.

Two, statically interesting, regions will be examined separately. The first comprises the two three-edge-supported slabs. The second comprises the cantilever and the two two-way slabs. The appropriate slabs are selected by pressing “Single” in the section “selection”.

The highest shears V_x varying from 23.1 to 38.8 kN, are developed at the free edge. V_x are somewhat smaller at the middle cross-section, varying from 21.1 to 36.4 kN. Shears V_y vary from 0.0 to 31.3 kN.

The above values refer to the section of the ‘Slab Results’ calculated from the integration of the stress resultants in distribution width 1.0 m. The respective values of the ‘FEM Results’ V_x vary from 27.7 to 40.8 kN at the free edges and from 21.1 to 36.6 kN at the middle cross-section. Shears V_y from 0.0 έως 31.9 kN. These differences are considerable only in the region of the free edges where there is a “crumple” as shown in the following 3D distribution of shears and especially in the side view of the 3D.

Shear forces [V_y] extend only in the regions of the middle supports.

One notices that the higher moments M_x, 16.9 kNm at the opening and -30.0 kNm at the support, are developed at the free edge. The values of M_x are lower at the middle cross-section, (13.8 kNm at the opening and -26.0 kNm at the support). Moments Μ_y vary from 0.0 to -17.4 kN. 

The above values refer to the ‘Slab Results’ section calculated from the integration of the stress resultants in distribution width of 1.0 m. The respective values of the ‘FEM Results’ for M_x are: at free edges, 17.0 at the opening and -30.5 kNm at the support, whereas at the middle cross-section, 13.9 at the opening and -26.2 kNm at the support. For moments Μ_y, in the middle cross-section values vary from 0.0 to -18.2 kNm.

The differences are insignificant

.

The distribution of bending moments [M_y] extend only in the regions of the top supports.

Shears V_x in the middle cross-sections of the two two-way slabs, vary from 18.6 to 33.6 kN compared to the almost same values 18.8 to 33.3 of the 1^st example. This means that the effect of the adjacent three-edge-supported slabs and the effect of the cantilever are insignificant at the middle region of the slab along x.

The same applies to the shears on the supports to the three-edge-supported slabs where their value 31.4 kN differ insignificantly from the respective shears of the 1^st example.

At the cantilever regions close to the three transverse linear supports of the two adjacent two-way slabs, shear forces are high due to concentration of extra loads transferred from the immediately adjacent slabs. This specific influence is well shown in the following figures, containing the detailed shear forces and bending moments distributions.

The extremely high shears in the region of the two-way slabs behind the cantilever is due to the negative load developed directly behind the cantilever in order to balance its strong moment Μ_y.

At the points of the slab (in this case of the cantilever) where fixed transverse support exist, numerous peaks are created, mainly for shears (in this case 130.8 and 138.6 kN) and secondarily for moments. These regions are forced to carry large part of the load of the adjacent slabs mainly near the supports. This is the reason why the occurrence of high shears shortly before and shortly after the support. However, these shears are taken into account in detail by considering their average values in a width, e.g. 1.0 m, which equals to 47.0 kN (‘Slab Results’).

Span moments of two-way slabs, are relatively low (M_x=8.9 kNm and M_y=4.9 kNm), compared to the respective values of the 1^st example (10.8 and 4.1 kNm).

At the common support, M_x,erm=-20.0 kNm  (the respective value of the 1^st example: -22.0 kNm), whereas at the border with the three-edge-supported slabs is M_y,erm=-17.4 kNm (the respective value of the 1^st example: -16.2 kNm).

It is concluded that the stress resultants on most of the area of the two-way slabs are similar to those of the 1^st example.

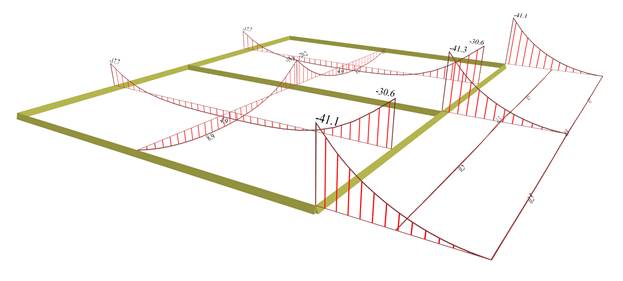
In the region of the continuity with the cantilever support moments are -30.6 kNm equal to almost isostatic moment of the cantilever. The higher moments of the cantilever - 41.0 kNm are the local peak moments, as shown in the next figure in the detailed distribution of the M_y due to the fixed transverse supports.

The positive peak moments forming a sharp “hole”, at the region of the support exactly behind the cantilever, results from the negative load created at this region by the cantilever’s moment.

Notice that the influence of the strong cantilever, both in negative and positive moments, decreases in a small distance from the cantilever support.

The insignificant differences in values of moments M_x between ‘FEM results’ and ‘Slab results’ are due to the relatively small curvature of the 3D moment diagram

M_x

.

Negative moments are developed on most of the region of the two-way slabs due to the strong cantilever support.

Along most of the support of the cantilever and the two-way slabs, bending moments have a value of-31.1 kNm whereas near the supports peak values occur within a width less than 1.0 m.

The maximum elastic deflection along the cantilever free edge varies from -2.75 mm at the mid-dle to -2.81 mm at both ends. The maximum deflections on the center spans are -0.64 mm.